The Solar Spectrum

The purpose of a solar simulator is to recreate the sunlight that we receive on Earth as closely as possible. To do this, we need to accurately replicate the solar spectrum. A spectrum shows the intensity of light as it varies with wavelength – so a solar spectrum tells us the measured intensity of light we receive from the sun at various wavelengths. The recognised reference spectra most often used as the standardised solar spectrum is the AM1.5 spectra. Classification of solar simulators is in part dependent on how accurately they can replicate this spectrum, so it is very important to consider when designing a solar simulator.
There are a number of factors that determine the shape and features of the solar spectrum. Sunlight starts its journey through complex nuclear reactions in the sun’s core and is then modified through interactions with the Earth’s atmosphere. As a result, replicating it presents a challenge. An overview of the most important factors affecting the light we receive are covered below.
Solar Spectrum Definition
The solar spectrum is the range of electromagnetic radiation emitted by the sun, extending from the ultraviolet to the infrared region. It is composed of photons with various wavelengths, which define the spectrum’s shape and intensity. It can be defined in terms of solar radiation or solar irradiance. Solar radiation is the direct emission of energy from the sun while solar irradiance is the amount of energy that reaches the Earth’s surface.
The solar spectrum can be divided into three main regions: the ultraviolet (UV), visible, and infrared (IR). The UV includes light with a wavelength shorter than 400 nanometers (nm). UV photons have energies ranging from approximately 3 to 5 electron Volts (eV). The visible region extends from 400 to 700 nm. This region contains photons with energies of 1.8 to 3 eV. Finally, the IR region comprises of light of wavelengths longer than 1000 nm, and its photons have energies between approximately 0.35 eV and 1.8 eV.
The intensity of the solar spectrum received on Earth is strongly dependent on the Earth-Sun distance, the angle at which the sun’s rays reach the Earth’s atmosphere, the weather, and the amount of air pollution. Solar cells, which use photovoltaic technology to convert solar radiation into electricity, are highly sensitive to the shape of the solar spectrum and the intensity of the radiation. Therefore, researchers and engineers working on solar cells must be knowledgeable about the solar spectrum in order to achieve optimal performance and efficiency. Therefore, the solar spectrum is an important phenomenon to consider when designing and working with solar cells.
Stellar Nucleosynthesis
The core of the Sun is undergoing a continuous process of nuclear fusion known as stellar nucleosynthesis. This occurs because the extreme heat at the core of the Sun (approximately 15 million Kelvin) is enough for the kinetic energy of atomic nuclei to overcome their electrostatic repulsion.
The proton-proton (or p-p) chain is the dominate fusion reaction in the Sun. This process converts hydrogen to helium. There are a few possible reaction routes, but the most common is the p-p 1 chain, which accounts for over 80% of the helium-4 created in the sun.
During the p-p 1 chain, two protons fuse together. This is an unstable product and will usually decay back to two protons. However, very occasionally, one of the protons will undergo β+ decay (emission of a positron and electron neutrino) and become a neutron, thereby forming a deuterium nucleus. When this occurs, the emitted positron will annihilate with an electron and release energy in the form of two gamma rays. The deuterium nucleus can go on to fuse with another proton to form a helium-3 nucleus and emit another gamma in the process.
The final stage of the process involves two helium-3 nuclei fusing to form a helium-4 nuclei and two protons. Along with the kinetic energy of the fusion products, the energy released as gamma rays from this stage of the process maintains the temperature of the core and allows stellar nucleosynthesis to continue.
Photons (gamma rays) produced in the core will migrate outwards. As they do, they interact with matter, exchange energy with their surroundings, and tend towards thermal equilibrium. While the core of the Sun can reach 15 million Kelvin, this temperature drops moving outwards. Simultaneously, pressure reduces, and fusion reactions become less prevalent. The surface of the Sun is approximately 5700 K.
Black-Body Radiation
A 'black-body' is a theoretical object in thermal equilibrium that absorbs all light that is incident upon it. Black-bodies have a single, well-defined temperature. The surface of the sun is a good approximation of these requirements. It will readily absorb incident electromagnetic radiation, while the photons near the surface of the Sun have a similar energy to that of the atomic nuclei. This is because they have been exchanging energy through Compton scattering.
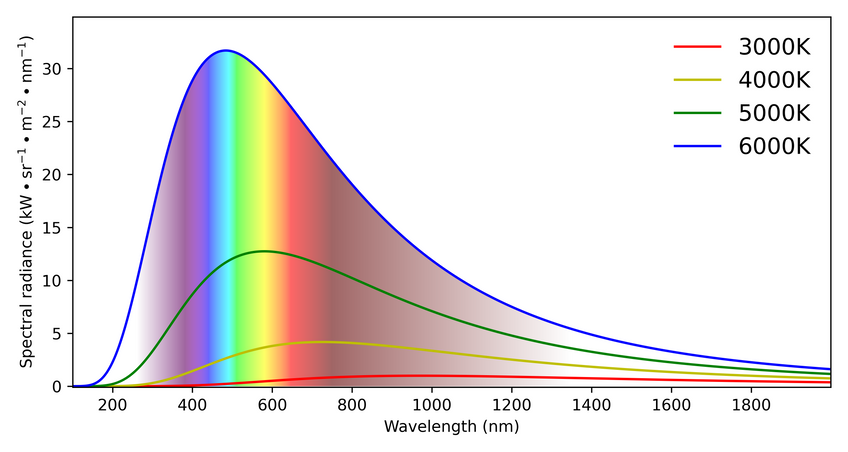
Black-body objects emit electromagnetic radiation. The spectrum of this radiation depends solely on the temperature of the object, as described by Planck's Law:
Here, B is spectral radiance, λ is wavelength, T is temperature, c is the speed of light, h is the Planck constant, and kB is the Boltzmann constant. The hotter the object, the 'bluer' the peak of the emission (shorter wavelength). Therefore, the more radiation it will emit.
What is spectral radiance?
Spectral radiance is a measure of the amount of power emitted from a unit area of surface into a unit solid angle over a given wavelength interval. The unit for spectral radiance is W. m−2.sr−1.nm−1.
The Sun's radiance can vary depending on solar activity (solar flares increase the amount of long-wave radio and short-wave X-ray emission compared to that expected from Planck's Law). However, with some noticeable deviations, it is approximated as a black-body with a temperature of ~5800 K.
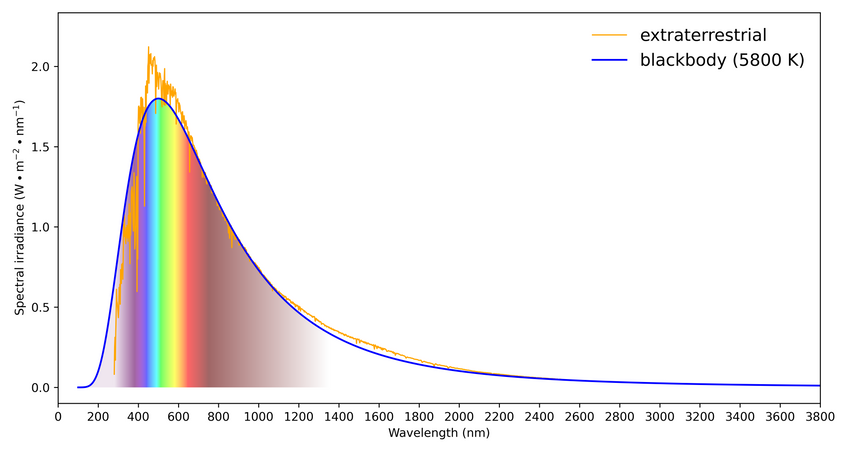
As you can see, the spectrum of the sun has a fine structure. This is due to the absorption of certain wavelengths of light by elements in the Sun’s atmosphere.
Solar Radiation
The average distance between the Earth and the Sun is approximately 150 million kilometres. This means that rays of sunlight are approximately parallel when they arrive at Earth.
The total solar power that reaches the top of the Earth's atmosphere is approximately 180 million GW (180 x 1015 W) with an extra-terrestrial irradiance of approximately 1350 W/m2. If you measured this here, the spectral shape would closely match the spectrum at surface of the Sun.
However, the amount of solar radiation which reaches Earth varies based on the time of year. This is due to variations in the Earth-Sun distance, which varies between approximately 147 million km at perihelion in January to 152 million km at aphelion in July. To a lesser extent, it also depends on the solar activity cycle; solar activity oscillates with an 11-year period, which results in changes in irradiance of approximately 0.1% over this period.
The Solar Zenith Angle
The angle at which the light intercepts the atmosphere is the governing factor in the irradiance at the top of the atmosphere. This angle is called the solar zenith angle, θs and is the angle between the sun's rays and local vertical. Due to the spherical shape of the Earth, the greater θs, the larger the area that the sun's rays are spread over.
The irradiance on the atmosphere for a particular solar zenith angle is given by the equation:
I0 is the irradiance on a surface perpendicular to the direction of incoming light.
The solar zenith angle depends on both location and time. Equatorial regions receive sunlight more perpendicularly than polar regions, so in general the further the latitude is from 0°, the lower the irradiance.
At different times of the year, different latitudes are directly facing the sun. At the solar equinoxes (March and September), the equator points directly at the sun (i.e., lies on the ecliptic plane) and receives normally incident sunlight. At the June and December solstices, the tropics of Cancer and Capricorn respectively point towards the sun and receive the highest irradiance.
The latitude that is directly facing the sun is given by the solar declination angle (the angle between the equatorial plane and the Sun's rays). This will vary approximately sinusoidally throughout the year and will be 0° at the equinoxes. This value reaches a maximum of +23.45° at the June solstice (corresponding to the latitude of the Tropic of Cancer) and a minimum of -23.45° at the winter solstice (corresponding to the latitude of the Tropic of Capricorn).
The solar noon is when the Sun's rays are most perpendicular to the local horizontal, this is why solar irradiation is at its maximum. However, it is important to note that the Sun’s rays can only be fully perpendicular at latitudes between the tropics. At times other than the solar noon (morning and evening), the Earth intercepts the Sun's rays at more oblique angles and hence the irradiation is lower.
The solar zenith angle is dependent on the latitude (Φ), declination (δ) and solar angle (ω) through the equation:
Solar Irradiance
The atmosphere is a particularly important factor in determining local terrestrial solar irradiance as it causes significant changes between the radiation at the top of the atmosphere and what reaches the ground.
Certain wavelengths are preferentially absorbed or scattered by atoms/molecules in the air. Absorption is the process whereby a photon of light transfers all its energy to an atom/molecule. It does this by promoting an electron to a higher energy level, such that the photon no longer exists. Consequently, its energy is effectively converted to heating the absorber (sometimes a photon can be remitted in a process called fluorescence, but this is rare in the atmosphere).
At ultraviolet wavelengths below 400 nm, O3 (ozone) is the primary absorber of radiation. Molecules in the atmosphere scatter incoming solar radiation into random directions. This is known as Rayleigh scattering and is responsible for the reduction in irradiance throughout the visible part of the spectrum.
Molecular oxygen (O2) is responsible for some absorption of light in the red and near infrared region (600 nm – 800 nm), as is water vapour (H2O), which also has strong absorption bands for light in the mid-infrared region up to 3000 nm. Carbon dioxide (CO2) begins to contribute to absorption of light above approximately 2500 nm.
Local effects, such as weather and pollution levels can further change the terrestrial spectrum.
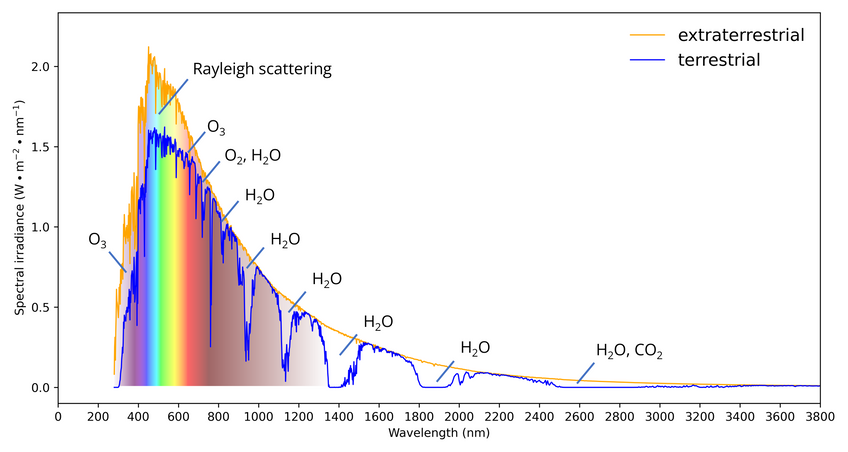
Atmospheric constituents can also scatter sunlight. Here, the photons are deflected from their original trajectory by molecules/particles rather than being absorbed. The two types of scattering that are most prevalent in the atmosphere are Mie scattering and Rayleigh scattering. Both are types of elastic scattering, so the wavelength (λ) of light is unchanged by the scattering process.
Rayleigh scattering occurs when the diameter (d) of the scatterer is much smaller than the wavelength of the light it is scattering. The intensity of Rayleigh scattering is proportional to λ-4, so it is far more efficient for short wavelengths (blue light) than long wavelengths (red/IR light). Rayleigh scattering can scatter light in all directions, with a slight prevalence to the forward and backward directions relative to the incoming light.
Gas molecules such as oxygen and nitrogen along with very small dust particles are largely responsible for Rayleigh scattering. This occurs mostly in the upper atmosphere. The preferential scattering of blue light by Rayleigh scattering is why the sky appears blue during the day and red at sunrise/sunset. Rayleigh scattering is also largely responsible for diffuse horizontal irradiance.
Mie scattering occurs when the diameter of the scatterer is equal to or larger than the wavelength of light. It preferentially scatters light in the forward direction, with larger particles scattering more heavily in favour of the forward direction. The intensity of Mie scattering is not heavily wavelength dependent. It occurs mostly in the lower atmosphere from water vapour and large dust particles/pollutants. Mie scattering is why clouds and fog appear white.
The more atmosphere the sunlight must pass through, the more scattering and absorption takes place. This is again related to the solar zenith angle, with more scattering and absorption occurring at larger solar zenith angles.
Measures of Irradiance
There are various common measures of solar irradiance at the Earth's surface, each of which is useful in different situations.
- Direct normal irradiance (DNI) is the solar irradiance measured on a unit area surface that is perpendicular to the direction of incoming sunlight (pointing directly at the sun). It only considers light that is not diverted from its initial direction, thus light that has not been scattered. It is useful for when you are considering solar concentrating or sun-tracking technologies.
- Diffuse horizontal irradiance (DHI) is irradiance that reaches a horizontal surface and excludes the DNI, thus is only the light that has been scattered by the atmosphere. It is useful if you are measuring local atmospheric levels such as pollution levels.
- Global horizonal irradiance (GHI) is the total irradiance incident on a horizontal surface and is the sum of the DNI (corrected for the solar zenith angle) and the DHI. The correction to the DNI is needed because it considers normally incident radiation rather than radiation falling on a horizontal plane.
GHI is the most useful value when considering non-tracking photovoltaic installations, and is the measure of irradiance that solar simulators try to recreate.
Solar Simulator

Learn More
Light can be measured either photometrically (only light visible to the human eye is considered) or radiometrically (also considers the energy in the invisible parts of the electromagnetic spectrum).
Read more... Solar Simulator Classification and Calibration
Solar Simulator Classification and Calibration
Solar simulators must be evaluated according to one of the three standards, and comply with the specifications set out within.
Read more...Contributing Authors
Edited by
Application Scientist
Reviewed by
Product Specialist